All Waves Are the Same (2015)
Color filters and fabric
234 x 129 cm
Unfolding the Tesseract Using Trees (2014)
Drawing, graphite on paper, plexiglass
50 x 60 cm
Mer sant (2015)
3D print of a Calabi-Yau manifold
15 x 14 cm
21x21x21x (2015)
Wood and glass
21 x 21 x 21 cm
Unfolding the Tesseract Using Cubes (2015)
Clip board, paper, 184 wooden cubes and lenticular plastic sheet
47 x 32 cm
Adding Dimensions (2014)
Video loop, sound loop and a plastic board
Dimensions variable
I Don’t Recognize Your Edge (2015)
Six plastic film cut-outs of Calabi-Yau manifolds
23 x 24 cm each
Curled Up (2015)
Birch bark, lenticular plastic sheets and rubber bands
Dimensions variable






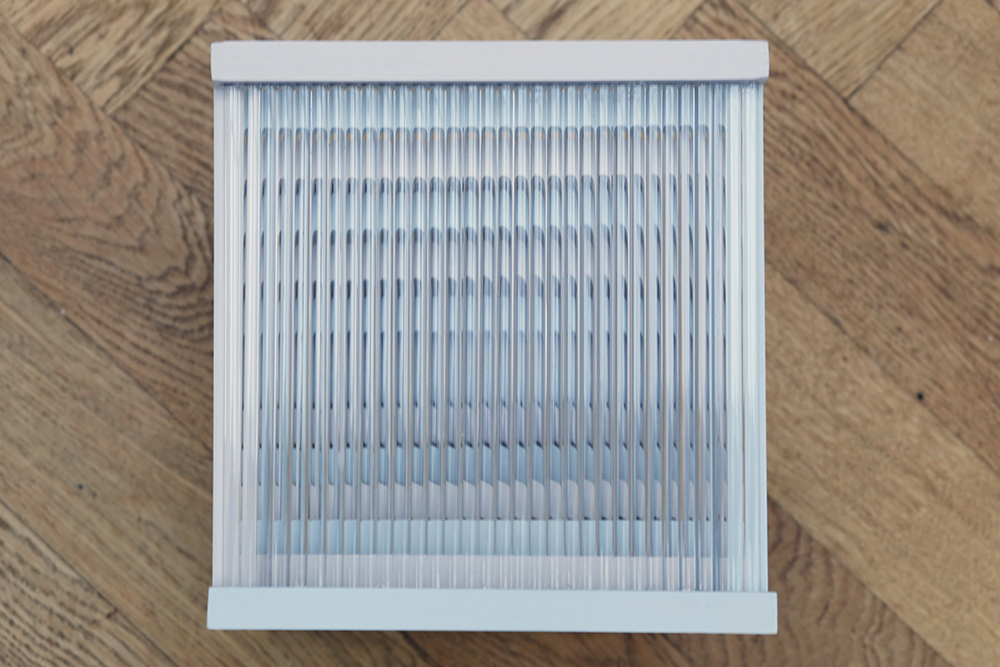



Documentation from the exhibition All Waves Are the Same at Galleri Pictura in Lund.
All Waves Are the Same
In semiotics, the first thing you encounter is a tree. You learn that the word ‘tree’ (the signifier) magically evokes an image of a tree in your mind, or demonstrates one in reality (the signified). From then on, this ‘tree’ serves to bring you back to basics, offering a comprehensible safety zone as you explore the labyrinth of language.
In quantum physics, the signifier and signified are blown apart. It may not be possible to conjure an image or explore a tangible reality for any of its terms. This field is not part of traditional science; its theories cannot be tested and the mere act of observation will alter any results.
Fascinated by the radical implications of the quantum, you patiently attempt to grasp its basic concepts; following along step by step, taking small breaths and jotting down notes, when suddenly the heated wooden floor falls out from beneath you and… logic fails. Why are there radio waves, micro waves, sound waves, electromagnetic waves, longitudinal waves, transverse waves, if really all waves are the same?
No one answers your question.
It’s suddenly 2016 and you are here. You may ask, “What the fuck is a Calabi-Yau manifold and why should I care?” Or on the other hand, you may sprain an eyebrow from trying to look away from the spellbinding hologram of an unfolding tesseract. Either way, if you get lost, just find your way back to the tree in the corner.
